Classification of Operators

Andrew Thangaraj

Aug-Nov 2020

Recap

Vector space \(V\) over a scalar field \(F= \mathbb{R}\) or \(\mathbb{C}\)
\(m\times n\) matrix A represents a linear map \(T:F^n\to F^m\)
- dim null \(T+\) dim range \(T=\) dim \(V\)
- Solution to \(Ax=b\) (if it exists): \(u+\) null\((A)\)
Four fundamental subspaces of a matrix
- Column space, row space, null space, left null space
Eigenvalue \(\lambda\) and Eigenvector \(v\): \(Tv=\lambda v\)
- There is a basis w.r.t. which a linear map is upper-triangular
- If there is a basis of eigenvectors, linear map is diagonal w.r.t. it
Inner products, norms, orthogonality and orthonormal basis
- There is an orthonormal basis w.r.t. which a linear map is upper-triangular
- Orthogonal projection: distance from a subspace
Adjoint of a linear map: \(\langle Tv,w\rangle=\langle v,T^*w\rangle\)
- null \(T=\) \((\)range \(T^*)^{\perp}\)
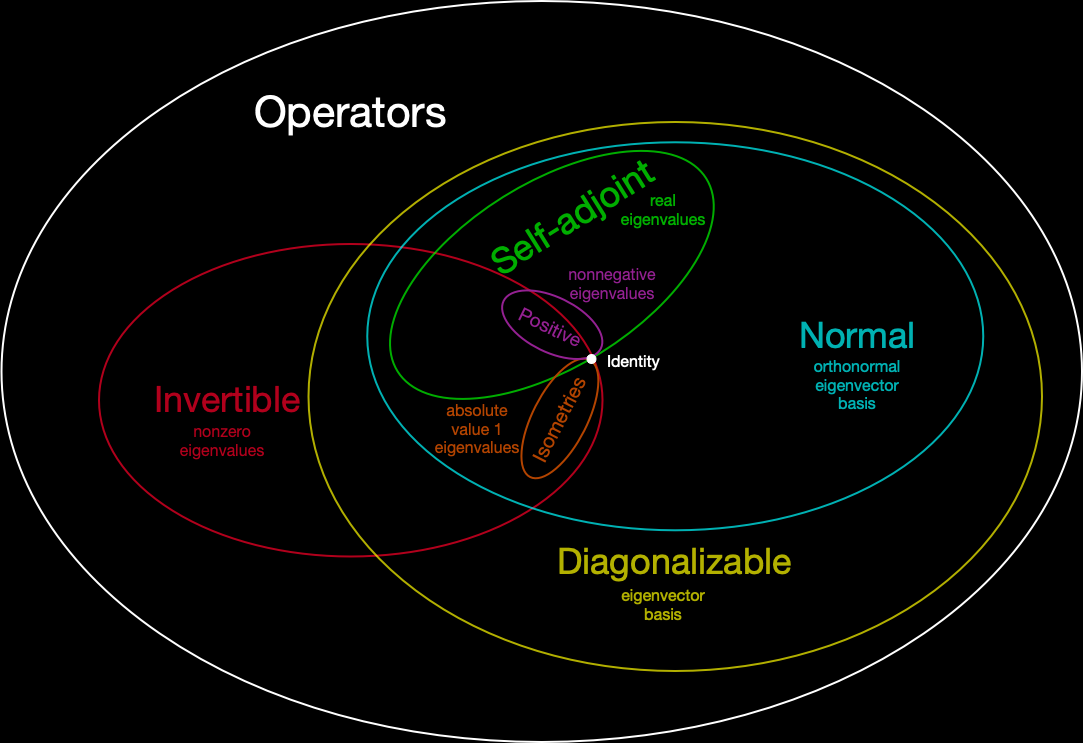
Self-adjoint: \(T=T^*\), Normal: \(TT^*=T^*T\)
Complex/real spectral theorem: \(T\) is normal/self-adjoint \(\leftrightarrow\) orthonormal basis of eigenvectors
Positive operators: self-adjoint with non-negative eigenvalues
Isometries: normal with absolute value 1 eigenvalues

\(V\): inner product space, dim \(V=n\), \(T:V\to V\)

Type	Property
Any	dim null \(T\) + dim range \(T = n\)
	null \(T=\) \((\)range \(T^*)^{\perp}\)
	dim range \(T=\) dim range \(T^*\)
	Upper-triangular matrix w.r.t. orthonormal basis
Invertible	dim null \(T=0\), dim range \(T=n\)
Diagonalizable	No special property
Normal	null \(T=\) null \(T^\), range \(T=\) range \(T^\)
	null \(T=\) \((\)range \(T)^{\perp}\)